
The image is of a large unit square with five smaller disjoint shaded squares contained entirely within it. The five smaller squares are congruent. Four of them are at each corner of the large square. The fifth is in the center, rotated diagonally, so the center of each of its sides is touched by the vertex from one of the other four squares. You are given that the common length for the five smaller congruent squares is (a-sqrt(2)) / b, where a and b are positive integers. What is the value of a + b?

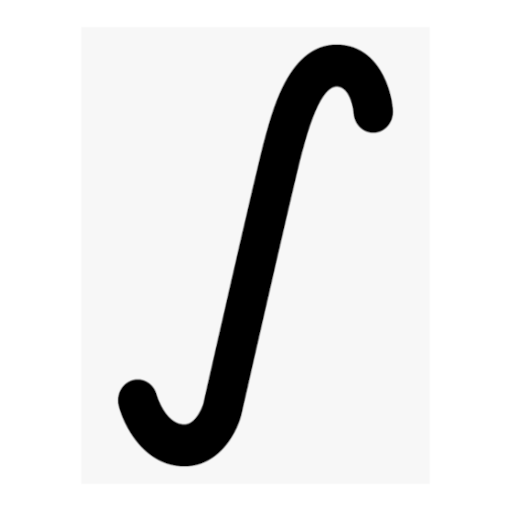
Since the meat of the problem is in the proof, I’ll mention the value of a + b is:
a + b =
11
further commentary
There are probably a few ways to prove this. I’ll mention at least one of those ways is both simple and short, within the grasp of your typical sophomore in high school.
I don’t plan to make this into a regular series like siriussmart’s, but I might occasionally post problems I come across that seemed interesting.